 Get premium membership
Get premium membership and access revision papers with marking schemes, video lessons and live classes.
Form 3 Mathematics Paper 2 End of Term 3 Exams 2021
Class: Form 3
Subject: Mathematics
Level: High School
Exam Category: Form 3 End Term 3 Exams
Document Type: Pdf
Views: 1054
Downloads: 92
Exam Summary
SECTION 1 (50 MARKS)
Attempt ALL the questions in the spaces provided
1. Make h the subject of the formula (3mks)
#E=1-pi sqrt((h-0.5)/(1-h)#
2. Solve for x in # log_3 (3^(x^2-13x+28)+2/9)=log_5 0.5# (3mks)
3. Given that
#8/(4-2sqrt3) = a + bsqrt3# and that a and b are rational numbers, find the values of a and b.(3mks)
4. Given that P varies directly as V and inversely as the cube root of R and that P = 12 when V = 3 and R = 2,
(i) Find an equation connecting P, V and R. (2 marks)
(ii) Find the percentage change in P if V is increased by 12% and R decreased by 36% (2marks)
5.The mass of two similar solid are 324g and 768g. Find the surface area of the smaller solid if the surface area of the bigger solid is 40cm².
6. The diameter of a circle has its ends with coordinates A(6, 10) and B(0, 2). Determine the equation of the circle giving your answer in the form #x^2 + y^2 + ax + by + c = 0#. (3 marks)
7. The cost of a matatu was sh. 950 000.It depreciated in value by 5% per year for the first 2 years and 15% per for the subsequent years. Calculate the value of the matatu after 5 years. (3marks)
8. Two circles centres A and B and radii 2.5cm and 1.5cm respectively. The distance between A and B is 7cm.
(a) Draw the required circles and construct the direct common tangents to the two circles. (2marks)
(b) Measure the length of the tangent (1 mark)
9. Use Logarithm tables to evaluate (4mks)
#sqrt((32.4times0.04352)/(cos 85.9°#
10. Solve the simultaneous equations: (4mks)
#4x-2y=6#
#x^2-xy=-4#
11. A tea dealer mixes two brands of tea, x and y to obtain 35kg of the mixture worth Ksh 62 per kg. If brand x is valued at Ksh. 68 per kg and brand y at Ksh.53 per kg. Calculate the ratio, in its simplest form, in which the brands x and y are mixed. (3mks)
12. Find the compound interest on Ksh. 21,000 in 3years at a Rate of 20% P.a. Compounded semiannually. (3mks)
13. A box contains 36 balls all of the same size and shape, if y of the balls are red, 19 are white and the rest are blue. A ball is picked from the box at random. If the probability that this ball is red is # 1/3# .Find
(a) The value of y (1mk)
(b) The probability that the ball picked is blue. (2mks)
14. Wekesa and Ndinda working together can cultivate a piece of land in 6 days. Wekesa alone can complete the work in 15days. After the two had worked for 4 days Wekesa withdrew the services. Find the time taken by Ndinda to complete the remaining work. (3mks)
15. The average of the first and fourth terms of a GP is 140. Given that the first term is 64. Find the common ratio. (3mks)
16. Eighteen labourers dig a ditch 80m long in 5 days. How long will it take 24 laborers to dig a ditch 64 m long? (3mks).
SECTION 2 (50 MARKS)
Answer ANY five questions from this section.
17. The #1^(st) , 7^(th), and 25^(th)# terms of an arithmetic progression are the first three consecutive terms of a geometrical progression. The 20th term of the arithmetic progression is 22. Find:
(i) The first term and the common difference of the arithmetic progression. (4marks)
(ii) The sum of the first 20 terms of the arithmetic progression. (2marks)
iii) The 7th term of the geometric progression. (2marks)
(iv). the sum of the first six terms of them geometric progression. (2marks)
18. A varies directly as M and inversely as the square root of Q. Given that A = 280,and M = 40 when Q = 16:
(a) find A when Q = 9 and M =36 (3marks)
(b) Find the value of M when A = 400 and Q = 0.64. (2marks)
(c) if Q is increased by 26%band M decreased by 20%, find the percentage change in A. (5 marks)
19) Mungai, Koskei and Kandie are participating in an athletics competition. The probability that Mungai, Koskei and Kandie complete the race are #3/5, 1/6 and 4/7# respectively. Find the probability that in a competition:
i) Only one of them completes the race (3marks)
ii) All the three complete the race (1mark)
iii) None of them completes the race (1mark)
iv) Two of them complete the race (3marks)
v) At least one completes the race (2marks)
20) The income tax rates in a certain year are as shown below
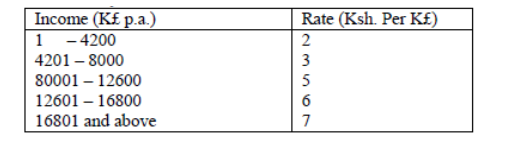
Omar pays sh. 4000 as PAYE per month. He has a monthly house allowance of Ksh. 10800 and is entitled to a personal relief of Ksh. 1200 per month. Determine
i).His gross tax per annum in Ksh. (2marks)
ii) His taxable income in K£ per annum (4marks)
iii) His basic salary in Ksh. Per month (2marks)
iv) His net salary per month (2marks)
21. A car hire company hire out cars such that there is a fixed charge and another part which varies with the distance covered. Taking C to stand for total cost, d for distance covered, k for fixed charge and t for charge per kilometer.
a) Express C in terms of k,tand d. (1mark)
b) Given that the total cost is 7000 when the distance is 200km and the total cost is 11000 when distance is 400km.
i) Find the values of k and t. (2marks)
ii) Find the equation connecting c,t,k and d. (1mark)
(c) Find the cost of hiring a car to area a distance of 500km. (2marks)
(d) Due to increase in fuel prices, the company increased the fixed charge by 20% and charge per kilometer by 10%:
i) Find the cost of hiring the car for 500km. (2marks)
ii) Find the percentage increase of hiring the car for the 500km. (2marks)
22. Purity bought a camera on hire purchase terms by paying a deposit of Ksh.7, 200 and cleared balance in 24 equal monthly installment each of ksh.1, 250.
a) Find the hire purchase price of the camera. (3marks)
b) The hire purchase price of the camera is 24%higher than the cash price. Find the cash price of the camera.
c) Eunice took a loan from a financial institution and bought the camera with cash. She repaid the end of the two years. Find the total interest paid by Eunice. (3marks)
d) A car is worth Ksh.800,000 when new. It depreciates by 20% every year. How much will it cost after five years. (2marks)
23. In the figure below, O is the centre of the circle. A, B, C and D are points on the circumference of the circle. A, O, X and C are points on a straight line. DE is a tangent to the circle at D. Angle BOC= 48° and angle CAD = 36°
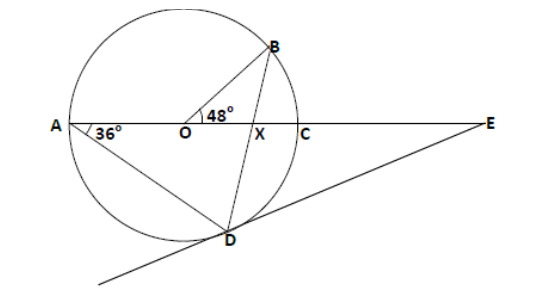
(a) Giving reasons or otherwise, find the value of the following angles:-
(i) Angle CBA (1 mk)
(ii) Angle BDE (2 mks)
(iii)Angle CED (3 mks)
(b) It is also given that AX = 12 cm, XC = 4 cm, DB = 14 cm and DE = 20 cm.
Calculate:
(i)DX (2 mks)
(ii)AE (2 mks)
24. The figure below shows triangle OAB in which OA =a and OB=b. M and N are points on OB and AB respectively such that #OM=1/3 OB and 5AN=2AB#. Lines AM and ON meets at P such that #OP =5/9 ON#.
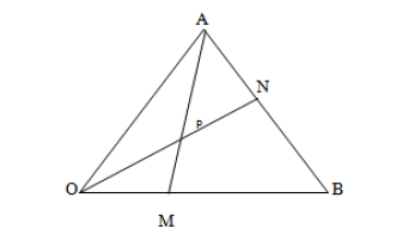
(a) Express the following vectors in terms of a and b
(i) AB (1 mk)
(ii) ON (2 mks)
(iii) AM (1 mk)
(b) Express AP and PM in terms of a and b and hence show the points A, P and M are collinear (5 mks)
(c) State the ratio AP: PM (1 mk)
More Examination Papers